What is the moment of inertia of particle a. Moment of inertia is the property of the body due to which it resists angular acceleration which is the sum of the products of the mass of each particle in the body with the square of its distance from the axis of rotation.

Ejsong Com Basic Physics Physics Formulas Mechanical Engineering
A 100 gram particle lies at point 11 a 200 gram particle lies at point -20 and a 300 gram particle lies at point 0-3.

. According to Newtons first law of motion an object that is stationary will remain stationary and an object that is in motion will continue to move in a straight line with the same velocity unless an external force is applied to it that is an object cant automatically. Just as linear motion has inertia so rotational motion has Moment of Inertia. The higher the moment of inertia the more resistant a body is to angular rotation.
The moment of inertia depends on the mass distribution and axis with respect to which we are calculating the moment of inertia and the circular ring and a particle have the same mass distribution that is the mass is concentrated at some distance. An axis of rotation has not been specified. Correct Part C Find the moment of inertia of particle a with respect to the x axis that is if the x axis is the axis of rotation the moment of inertia of.
Moment of Inertia I Σ m i r i 2. Basically for any rotating object the moment of inertia can be calculated by taking the distance of each particle from the axis of rotation r in the equation squaring that value thats the r 2 term and multiplying it times the mass of that. An axis of rotation has not been specified.
Moment of inertia can be defined as a quantity that expresses a bodys tendency to resist angular acceleration which is equal to the sum of the products of the mass of each particle in the body with the square of its distance from the axis of rotation. The moment of inertia of a particle is the product of the mass of the particle m and the square of the distance between particles with the axis of rotation r2. Moment of inertia of a particle about an axis of rotation that doesnt pass through it is expressed a product of its mass and the square of its distance from the rotation axis.
That measurement is calculated based upon the distribution of mass within the object and the position of the axis meaning that the same. The moment of inertia I is calculated by multiplying the mass of each particle of matter in a particular body by the square of its distance from the axis. MOI varies depending on the axis that is chosen.
Find the moment of inertia of the particle described in the problem introduction with respect to the axis about which it is rotating. We note that the moment of inertia of a single point particle about a fixed axis is simply latexmr2latex with r being the distance from the point particle to the axis of rotation. This arises from the fact that although mass is always conserved the moment of inertia in general is not.
A particle is located at position r relative to its axis of rotation. The general formula represents the most basic conceptual understanding of the moment of inertia. For now we leave the expression in summation form representing the moment of inertia of a system of point particles rotating about a fixed axis.
Moment of Inertia Formula. Find the moment of inertia Ix of particle a with respect to the x axis that is if the x axis is the axis of rotation the moment of inertia Iy of particle a with respect to the y axis and the moment of inertia Iz of particle a with respect to the z axis the axis that passes through the origin perpendicular to both the xand y axes. A uniform bar has two small balls glued to its ends.
However both depend on the following factors. The mass moment of inertia depends on the distribution of. The moment of inertia of a particle or a system of particles is given by.
This is the best answer based on feedback and ratings. Therefore given a particles mass and its distance from an axis of rotation we can calculate its mome. Before discussing the moment of inertia of a rigid object first study the moment of inertia of a particle.
So the use of the term particle is only to facilitate discussion of the motion where. It mainly depends on the distribution of mass around an axis of rotation. The moment of inertia is often calculated for a system of particles or a continuous body taken as a whole one particle.
Moment of Particle Inertia. Of the system found in Part D find the total kinetic energy of the system. 4 Three particles lie in the x-y plane.
All distances are measured in meters. Find the moment of inertia Ihoop of a hoop of radius r and mass m with respect to an axis perpendicular to. 100 42 ratings i m9rr or mrr.
Equation 6 is used to determine the moment. When a force F is applied to the particle. Thus they have the same expression for moment of inertia.
The SI unit of moment of inertia is kg m 2. That is to say it measures how difficult it would be to change an objects current rotational speed. So I know this.
The moment of inertia otherwise known as the mass moment of inertia angular mass second moment of mass or most accurately rotational inertia of a rigid body is a quantity that determines the torque needed for a desired angular acceleration about a rotational axis akin to how mass determines the force needed for a desired accelerationIt depends on the bodys. What is the moment of inertia of particle a. A body is usually made from several small particles forming the entire mass.
Its formula is given by I Lω where I denotes the inertia L denotes the angular. Where m Sum of the product of the mass. There is actually no set size limit for said particles.
What is the moment of inertia for this system rotating in the x-y plane around the origin. In this case do not think of the particle as a very small object. When computing angular momentum for a rigid body the MI is analogous to mass in linear motion.
Remember that both particles rotate about the y axis. The moment of inertia of an object is a calculated measure for a rigid body that is undergoing rotational motion around a fixed axis. Answer 1 of 2.
Moment of Inertia. Proof of the equivalence of definitions. The bar is 200 m long and with mass 400 kg while the balls each have mass 0500 kg and can be treated as point masses.
I m r2 Equation 6 I moment of inertia of particle m mass of the particle r distance between particle with the axis of rotation. It depends upon the density of the material since the moment of inertia is related to the mass of the particle and mass is dependent on the density of a particle. The moment of inertia or mass moment of inertia is a scalar quantity that measures a rotating bodys resistance to rotation.
Moment of inertia is usually specified with respect to a chosen axis of rotation. Moment of Inertia is also known as the angular mass or rotational inertia.
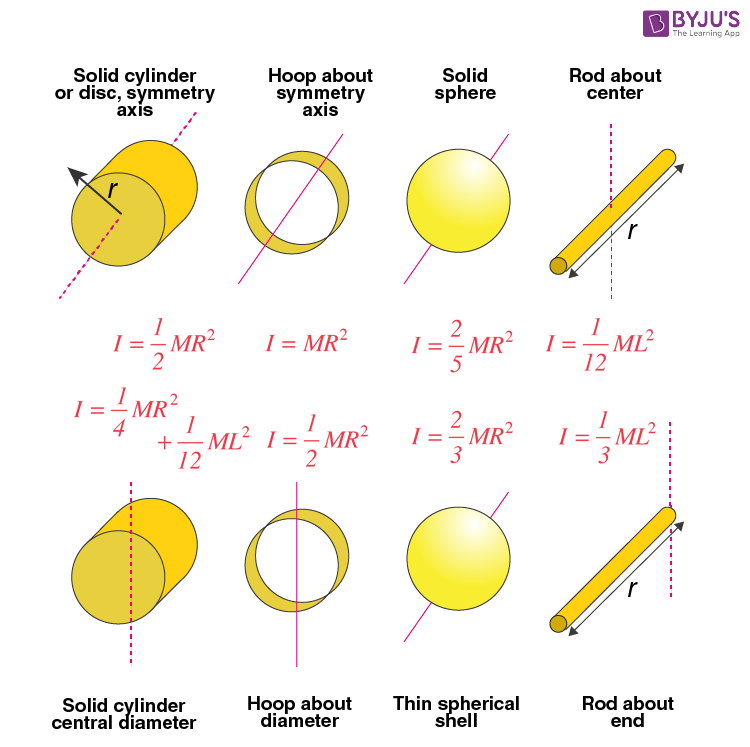
Moment Of Inertia Formulas Moi Of Objects Solved Examples
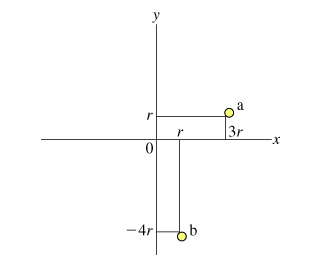
Solved What Is The Moment Of Inertia Of Particle A A Chegg Com

What Is The Moment Of Inertia Ia Of Particle A Fornoob

10 4 Moment Of Inertia And Rotational Kinetic Energy University Physics Volume 1
0 Comments